New Progress in the Basic Research for Topological Quantum Computing: Zero-Energy Bound States in the High-Temperature Superconductors at Two-Dimensional Limit
Result of the Month
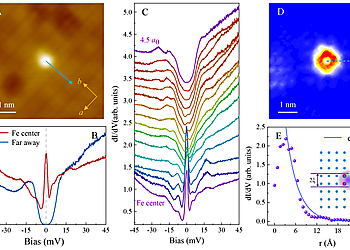
By using the MBE technique, our one-unit-cell (1-UC) FeSe and FeTe0.5Se0.5 films were well prepared with atomically flat surfaces at both mesoscopic and microscopic scales. The Fe atoms were deposited on the 1-UC FeSe and FeTe0.5Se0.5 surfaces at ~143–155 K at an ultralow coverage for the formation of individual adatoms (e.g. Fig. 1A for 1-UC FeSe). Spectroscopically, the zero-energy bound state (ZEBS) modulated by adsorbate-substrate interaction is induced by the interstitial Fe adatom, which appears as a zero-bias conductance peak (ZBCP) in the tunneling spectrum and is exceptionally sharp with a peak-to-dip dI/dV ratio of ~3 (Fig. 1B). The spatial evolution of the tunneling spectra along a linecut departing from the adatom is presented in Fig. 1C. As moving away from the adatom center, the zero-bias signal drops abruptly but remains a single peak before becoming unidentifiable. The unsplitting behavior of the ZEBS here is noteworthy and reminiscent of the unsplit Majorana-like ZEBS off magnetic-vortex center in superconducting topological surface states. To directly visualize the ZEBS distribution in space, a dI/dV mapping for the Fe-adatom topography in Fig. 1A was measured at 0 mV (Fig. 1D). Enhanced feature intimately bounded to the adatom edge was found in the ZEBS pattern, which correlates with the phase decoherence by the Fe adatom. For a more quantitative analysis, the linecut profiles starting from the adatom center were extracted from Fig. 1D and one of them, L, is exemplified in Fig. 1E. The exponential fitting of L yields a decay length ξ of 3.4 Å, which is nearly one order of magnitude smaller than the superconducting coherence length (2.45 nm).
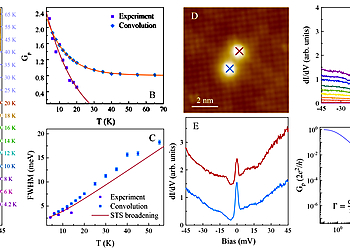
Basically, the Fe adatoms deposited on 1-UC FeSe and FeTe0.5Se0.5 films share nearly the same spectroscopic results. We take 1-UC FeTe0.5Se0.5 as an example. The ZBCP in the experimental spectrum of 1-UC FeTe0.5Se0.5 disappears at a temperature of 20 K that is still well below superconducting critical temperature Tc, in contrast to the thermally convoluted ZEBS spectrum with assumed impurity-state origin (Fig. 2, A and B). For an Fe-adatom dimer, the ZEBS spectrum remains singly peaked (Fig. 2, D and E). Both the premature thermal melting and the spectral unsplitting against local magnetic-exchange field for the detected ZEBS in 1-UC FeTe0.5Se0.5 emphasize the difficulty in describing the ZEBS in terms of the conventional impurity-scattering state. Furthermore, the full width at half maximum (FWHM) result is consistent with the intrinsically single-peak nature of the detected ZBCP (Fig. 2C) as expected for Majorana zero modes (MZMs). Most intriguingly, the experimentally detected ZEBS (4.2 K) exhibits rather robust existence for a wide range of the tunneling-barrier conductance GN over several orders of magnitude (Fig. 2F). By detailed scaling analysis based on ZBCP (Eq. 1; ) for MZMs, the GN-dependent ZBCs Gp extracted from the experimental ZEBS spectra in Fig. 2F is quantitatively described by the universal scaling of Majorana ZBCP (Fig. 2G), yielding η = 0.35 comparable with that (0.25) obtained in 1-UC FeSe. All above experimental observations resemble phenomenologically the spectroscopic signatures of the MZM. The reproducibility of the phenomenological MZM features in different 1-UC iron chalcogenides possibly suggests a common topologically nontrivial origin of the detected ZEBSs. In light of the possible topological phases and spin-orbital coupling induced spin-triplet pairing components in 1-UC FeSe and Fe(Te,Se), the hidden mechanism responsible for the observed ZEBSs may be the quantum anomalous vortices nucleated at the magnetic Fe adatoms in 2D topological superconductors. The experimental systems described here integrate nearly all the desired ingredients for feasibly realizing and manipulating the MZM-like ZEBS: the significantly increased , the unnecessity of external magnetic field for inducing the ZEBS, the ultrashort ZEBS decay length and the technically feasible STM manipulation of adatoms further push the experimental systems towards applicable quantum-functionality electronics.
Authors
Chaofei Liu1, Cheng Chen1, Xiaoqiang Liu1, Ziqiao Wang1, Yi Liu1, Shusen Ye1, Ziqiang Wang2, Jiangping Hu3, Jian Wang1
Institutes
1) International Center for Quantum Materials, School of Physics, Peking University
2) Department of Physics, Boston College
Name and email of corresponding author
Prof. Jian Wang, email: jianwangphysics@pku.edu.cn
Publication
Science Advances 6: eaax7547 (2020)
URL of Journal
https://advances.sciencemag.org/content/6/13/eaax7547